communicationsraka.blogg.se

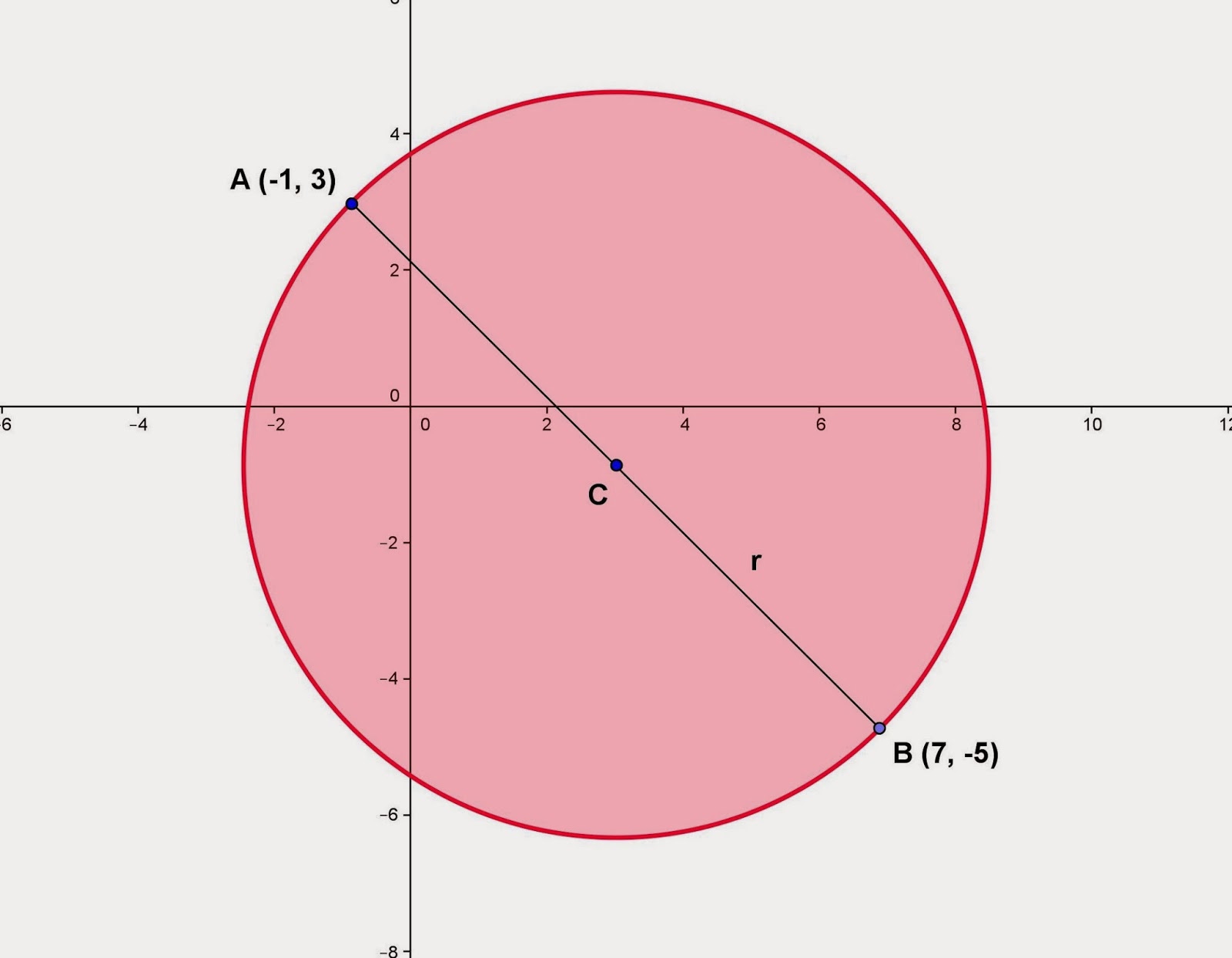
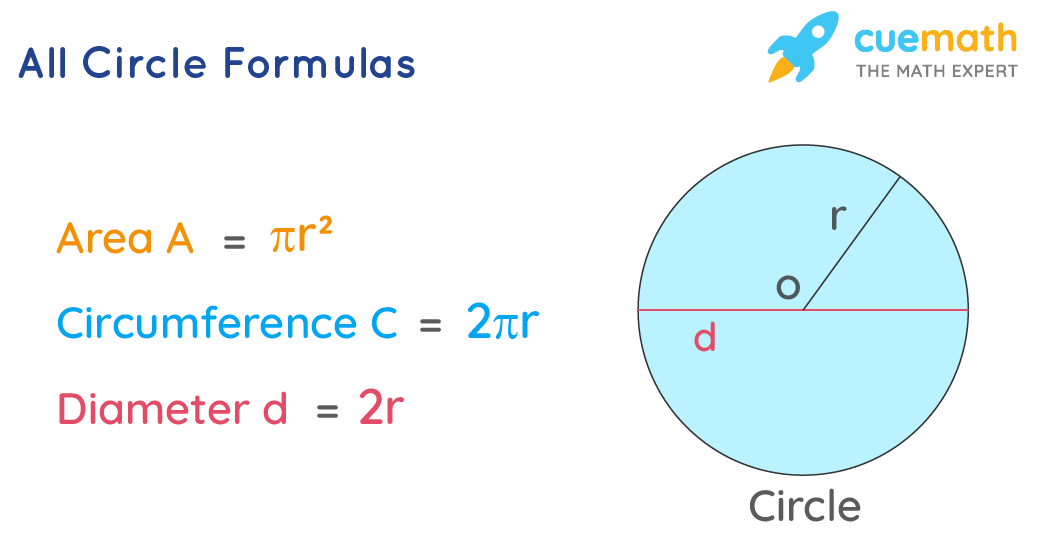
This is equivalent to the usual formula saying that the circumference of a circle with radius $r$ is $2\pi r$. An example of such a triangle (taken from a regular hexagon) is pictured below:Īs noted in the picture, these isosceles triangles have two sides of length $r$, the radius of the circle, and the third side $\overline$. A regular polygon with $n$ sides can be decomposed into $n$ isosceles triangles by drawing line segments connecting the center of the circle to the $n$ vertices of the polygon. The first solution requires a general understanding of similarity of shapes while the second requires knowledge of similarity specific to triangles.Īn alternative argument using trigonometric ratios provides a formula for the circumference of a regular polygon with $n \geq 3$ sides inscribed in a circle. High school students will know that the circumference of a circle of radius $r$ is $2 \pi r$ and therefore the goal of this task is to help them understand this formula from the point of view of similarity. This former approach is simpler but the latter has the advantage of leading into an argument for calculating the area of a circle. Two different approaches are provided, one using the fact that all circles are similar and a second using similar triangles. In order to show that the ratio of circumference to diameter does not depend on the size of the circle, a similarity argument is required. It is important to note in this task that the definition of $\pi$ already involves the circumference of a circle, a particular circle. This well known formula is taken up here from the point of view of similarity. Thus the area of one of the combined triangle whose center angle is `theta` is defined as 2 * 1/2 * base * 1/2 * height.The circumference of a circle of radius $r$ is $2\pi r$. The area of the segment can be seen graphically to be the difference between the area of the sector (defined by r and `theta` in the equation above) and the the area of the triangle defined by r and `theta` above - or more properly the summation of the area of the two right triangles with sides r, a, and b in the left picture. The simplified form of the formula for the area of the sector depends on whether the units used are degrees or radians. This equations area is derived in the equation, " Circle -area of sector" To find the area of the arc segment, we first find the area of the arc sector, shown in red in the second image on the right. The area of the arc segment is defined by the angle `theta` and the circle's radius, r. The area described in red in the first image on the left below is the area of the arc segment of of a circle. Area of Annulus given the inner radius ( r) and outer radius ( R).Area of Cirlce Circumscribing a triangle given three sides ( a,b,c) of the triangle.Area of Circle Incribed within a triangle given three sides ( a,b,c) of the triangle.Area of Arc Segment given radius ( r) and the depth ( h) into the circle.Area of Arc Segment given the radius ( r) and angle (`theta`).

Area of a Sector (wedge, pie piece) based on radius ( r) and angle (`theta`).

Area based on circumference: A = `pi (c/(2pi))^2`.

Area based on diameter: A = `pi (d/2)^2`.

However this can be automatically converted to other area units such as square feet or acres via the pull-down menu: The calculator returns the area in square meters. `A=1/2 * r^2 * (2 cos^-1((r-h)/r)- sin(2 cos^-1((r-h)/r))`įor the area of different parts or segments of a circle, the following calculators will help. (See figure) The following equation calculates the area using r and h: (Click on formula for solver) If θ is unknown, the same area can be calculated if the depth ( h) from the edge of the circle toward the center is known.

A = f(r,h) - Compute the area of an Arc Segment of a Circle based on the radius ( r) and depth ( h).

Start now: Explore our additional Mathematics resources. A ( d 2 4) where d is the length of the diameter. To calculate the area of a circle using the diameter, we have to substitute this equivalence in the area formula: A r 2. However the user can automatically convert the output units to numerous other compatible units via the pull-down menu. This distance is equal to twice the radius, that is, we have d 2 r or equivalently r d 2.

( θ) - This is the angle defining the arc.Īrea of an Arc Segment of a Circle (A): The calculator returns the area (A) in square meters.

( r) - This is the radius of the circle.

INSTRUCTIONS: Choose units and enter the following:

(θ - sin(θ)), computes the area defined by A = f(r,θ) A = f(r,h) an arc and the chord connecting the ends of the arc (see blue area of diagram).

The Area of an Arc Segment of a Circle formula, A = ½